Tricky Sudoku puzzles to solve
Get some help and solution ideas from these difficult to solve puzzles...
Here are some hard puzzles that have proved difficult to solve.
Contributors have uploaded puzzles for you to look at and help them to solve.
Any comments ? Click here to contributeThere does not seem any place to allocate.
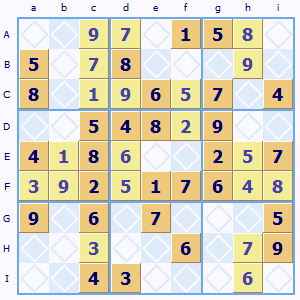
Has anyone any suggestions on how best to tackle this one?
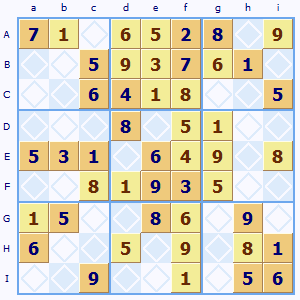
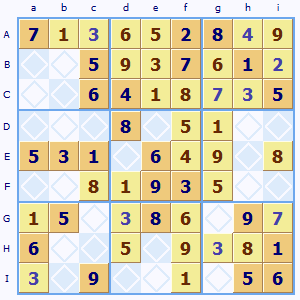
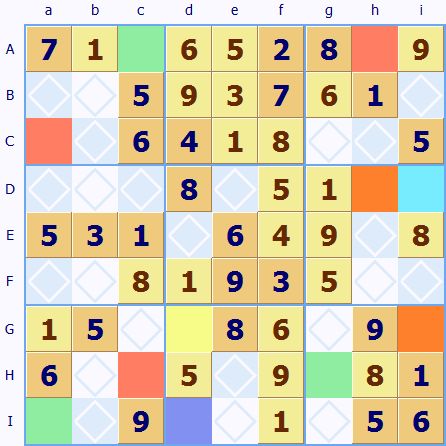
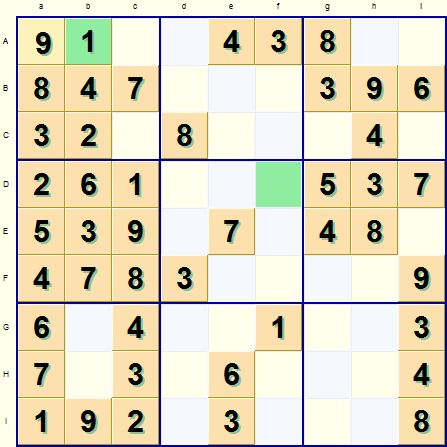
Any comments ? Click here to contributeAfter putting the 3 in Ac you can now put 4 in Ah.
Then in region Bg the square Bi can only take one value a 2.
Now have lots of twins that aren't helpful and it is the 3s that are worth loking at.
In row H there is only one place a 3 can go Hg and in region Ga the 3 must go in Ia.
That then forces a 3 in Gd and a 3 in Ch and the last 3 in Di. That is all the 3s allocated.
Putting a 7 in Cg completes the region and that 7 forces a 7 in Gi.
With only 25 squares left to solve that's probably solved it more or less.
Any comments ? Click here to contributeHi "Rob Puzzle"
In the 1st line of your reply:
"After putting the 3 in Ac you can now put 4 in Ah."
I don't see how you can declare that a 3 goes into Ac. For instance a 3 can also go into Ca in Region Aa. What is the basis for deciding that?
Any comments ? Click here to contributeI agree it looks like this is just an either-or guess. If you follow through with a '4' it does end up insolvable so there is no doubt the '3' in AC was correct/
Any comments ? Click here to contributeI must admit I can't remember!
Looking at now I can't see why I thought a '3' in Ac.
Perhaps it will come to me in time, I don't think it was a guess...
Any comments ? Click here to contributeYou are right there is no easy square to solve.
There is only one 2 allocated anywhere, and only two 4s and 7s.
To get you started try this strategy.
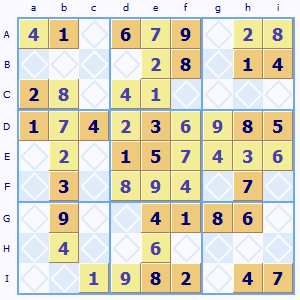
Concentrate on region Aa and Row C. A 3 must either be in Ac or Ca and a 9 in Ca or Cb. But 2 can only occur Ca or Cb in this region so this means a twin has been found and 3 can not occur in Ca it must be in Ac.
That should unlock things a fair bit.
Any comments ? Click here to contributeI agree that is the best way. But you don't say why you chose it in the first place.
How I'd approach it is to flick through using the possibilities bar in SudokuDragon. Go through all the numbers 1 to 9 using the ALT+number keyboard shortcut.
You'll see all 1s and 5s are allocated and that 6,8 and 9 all have a very symmetric pattern (often tricky to solve). There are lots of places that 2,4 and 7 can be allocated - far too many to think through easily. The only remaining number 3 has relatively few squares and a non-symmetric pattern. That is why I'd home in on looking at where 3s could be allocated.
Any comments ? Click here to contributeHi robert you said "But 2 can only occur Ca or Cb in this region so this means a twin has been found and 3 can not occur in Ca it must be in Ac" I understand the logic but
why isn't 2 possible in Ba and Bb? I see they aren't after 1 3 in Ac and a 4 in Ah leaving the 3 in Bi , but how did you eliminate then as candidates?
Thanks Steve
Any comments ? Click here to contributeYou are right Steve, I do not see how 'Rob Puzzle' can claim Ba and Bb can't take a 2.
I hope he wil come back and explain himself.
Any comments ? Click here to contributeIt needed me quite a bit of time but i found a reason for putting one more 3. If you search some pair for the number 3 you'll end in this configuration. Now if the 3 was in Dh so it would be also in all of the green squares and in the yellow as well. It should be also in the other orange square (Di) but this would end in a conflict so the number 3 has to be in Di. Unfortunately it didn't give so much help to me so far, but i'm still working on it.
Any comments ? Click here to contributeWell.. I can't even put one number..
Any comments ? Click here to contributeI think the original response was right - there is no way to solve this one but trial and error, which makes it in my view an invalid Sudoku.
Any comments ? Click here to contributeI got through 77 Sudoku puzzles in 'The Ultimate Sudoku Challenge' and hit one in the Section called 'Beware! Very Challenging' and I got stuck.
I included it (I think). Can anybody just get the next possible slot? and tell me how? I checked all my slots so far, and the programs says they are ok.
Thanks
CBK
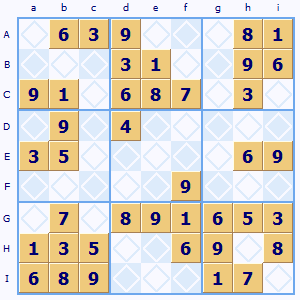
Any comments ? Click here to contributeI believe you are right this is a tricky one.
I can't see any way but to use trial and error.
Fortunately both the '1's and '2's have very limited possibilities - there is only one correct and one incorrect allocation possible. So I think all you need to do is to try allocating 1 in Db or Dc and follow the logic through. It will either solve or lead to a contradiction.
This probably makes it a 'bad' puzzle (see the discussion on Labyrinth) so don't be too hard on yourself.
Any comments ? Click here to contributeHello,
Well I solved it. Like I said in title, I got stuck a long time ago, and just decided to try again after many failures. The Sudoku Dragon program actually helped me, because I found a new strategy from one of its randomly created puzzles.
I will detail the solution here. Unfortunately I don't know how to get puzzles to appear here with those nice labels for row and Column, but I will use that system anyway.
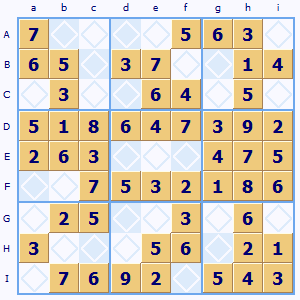
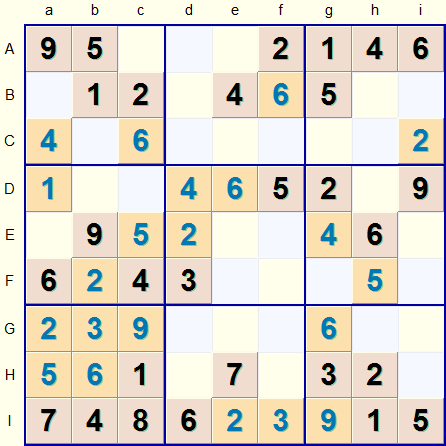
The Key item is the three squares Eb, Ec, and Ed. If you look at those squares, there are only 3 possible choices 5,7,9. Even though 5, 7, 9 appear in the other possible slots for row E, the fact that those 3 are limited to three slots is important.
For example, if you try to determine if a 5, 7, or 9 could be a possible solution to any slot other that Eb, Ec, and Ed you will get a contraction. So if Ei were 5, then Eb could not be 5 and would have to be 9, but that would make Ec 7, and then the only possible options for Ed would be a 9 or 7, which obviously have been taken up already. Same for 7,9.
This means that you can remove the options 5,7,9 from All E's other than Eb,Ec,Ed. This leaves another interesting thing, because now Eg can only be 4 or 6. If you look down g column you see that Gg can also only be 4 or 6. This means that you can remove 4 and 6 from all other squares in the g column, because 4,6 are limited to these to slots.
So if you remove 4,6 from column g, the three slots Ag,Bg,Cg in the Ga box now have the same property like Eb,Ec,Ed. There are three options 3,7,8 in three slots where if you were to place any of them else in the box there would be a contraction or impossible slot. So 3,7 can be removed from rest of the box, the 8 was stuck in g already.
Now this can puzzle can be solved. Whew!
CBK
Any comments ? Click here to contributeI know I can eliminate the possibility of 1 from Ca... but this isn't getting me anywhere. I'm completely stumped.
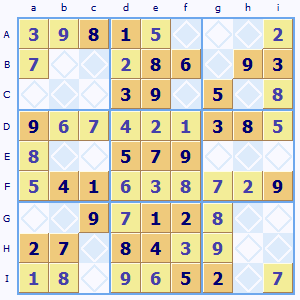
Any comments ? Click here to contributeWell, yes this was quite a challenge, thanks!
You are right 1 can not go in Ca because of the 1s in column c.
If you now look at the 4s you'll see they make an interesting pattern - only in columns a;b and c.
I think this is a Swordfish as if a 4 went in Ab a 4 must go in Fa, similarly if (the only alternative) the 4 went in Ac a 4 must go in Hb and so the 4 in region Da must be again in Fa. So Fa is 4 either way it cant go in Fb.
That also mean a 4 must go in Gd and so Hd must be 7 (only square in column Gd). Then a 9 in Fb to complete the region.
Any comments ? Click here to contributeSorry Alexander, your Swordfish is wrong.
Indeed if you look at the alternate pairs you will see that a 4 can not go in Hb. Even if it is Swordfish it doesn't help at all.
I solved this by looking at naked pairs. The square Ae is key, '1' can not go here because of the 1;2 naked pair in column d so there is now a naked pair 8;9 in row A. If you then exclude 8 and 9 from elsewhere in the row you are left with only a 4 in square Ab and so a 4 goes in there. The remaining squares are easy.
Any comments ? Click here to contributeI loaded the puzzle to my personal sudoku cruncher. There is an obvious two-number ambiguity in row I... 1,8
I placed an 8 in cell If. That exposed several singularities around the matrix. The puzzle was totally solved in a few minutes.
Any comments ? Click here to contributeI'm completely stumped on this puzzle, and hoping someone can give me some direction.
I've already looked and identified any possible locked candidates (I think), and no more numbers can be eliminated from the naked pairs that are in the puzzle.
There are 3 X-wings that give no help (7's on rows D,I), (5's rows H,I), (9's, row EI).
I've marked down each line and instance of a pair so I could more easily look for a swordfish, and I was not able to find one in this puzzle, nor could I find an X-Y Wing.
Without starting at a random point and guessing one number then solving, is there another way to solve this one? Thanks so much for your help! Ray
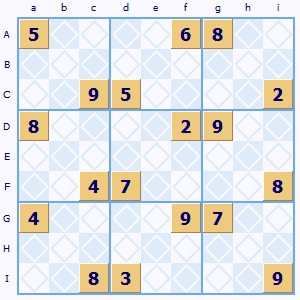
Any comments ? Click here to contributeMust admit to being a little but stuck with this one too. You are not alone.
May be a 'guess' is the only way forward. Frustrating.
Any comments ? Click here to contributeI had another good look and I now think there is a 'hidden' X-Y Wing or Hook. In square He only 4;5 is possible (2 excluded by naked [1,2] twin in region Gd) and
Hb only 5;8 (2 excluded by another naked [1,2] twin in row H). This forms the stem of my Hook, I now need a 4;8 square and that is in Gf. Using the contorted X-Y logic of the hook strategy that knocks out a 8 in square Gb. That leaves only 2 as the options for
this square Gb and I think that more or less wraps it up.
Any comments ? Click here to contributeI am not able to solve this puzzle using logic. Maybe someone can show me how.
Any comments ? Click here to contributeSorry I can't see anyway forward without 'guessing'. It does look like one of those puzzles that needs trial and error which I hate!
Any comments ? Click here to contributeYou need to look at Alternate Pairs to solve this one and even then it is a struggle.
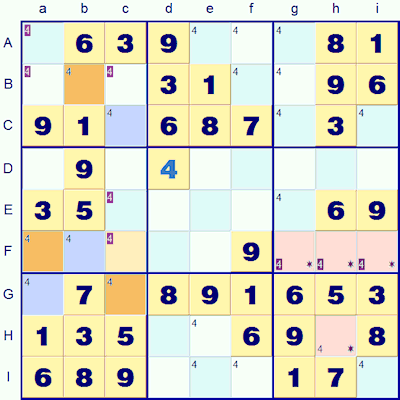
Look at where the 4s can go. Sudoku Dragon has been able to find that many of the 4s can be excluded and this helps a lot. The 4s form an interesting pattern of pairs. In Region Bb color Bb as orange then Cc is blue, but this is linked to Gc so that is orange and in row G this is linked to Ga in blue which links to Fa in orange . But Bb also links to Fb so this is blue. There are now an orange and blue square in row F so that discounts the 4s as possibilities in Fg; Fh and Fi (pink). Now that forces a 4 into square Hh as that now is the only square left in column h that can take a 4. And that I think is the crucial step.
Any comments ? Click here to contributeDid you want the solution to this puzzle or just the next step?
I developed a tool using Microsoft Excel that facilitates parallel analysis on sudoku puzzles. It amounts to a real time ’pencil mark‘ matrix for cells, rows, columns and 3x3 sectors. It also identifies forced singularities (only one possible number) for cells.
Using this tool, I solved the puzzle in about 5 minutes. The key was a 4 in cell Hh.
Any comments ? Click here to contributeI am stumped and there are no hints available. Can you tell me what I should do next ?
Any comments ? Click here to contributeLooking at the puzzle and SudokuDragon does not currently help with this, there are some possibilities that can be discounted.
Most are related to 'chains'. If you look at the Strategy page on this site you'll find something about them under 'General permutation rule'
basically if you have a group of {1,4} {4,7} and {4,7,8} and these are the only occurrences of 1,4 and 7 in the group you can discount the '8' as a possibility.
Here goes then. In column c there is a chain 23,34,45,56,62 this means 4 in Cc can be discounted.
In row A there is a chain 46,67,74 so 4 as a possibility in Ah can be knocked out.
In row H there is a chain 15,56,61 so 6 as a possibility in Hh can be knocked out.
Is this helping at all?
I think there are a few more to find using the same strategy.
In
Any comments ? Click here to contributeI received this puzzle from a friend. When I got stuck, I plugged it into SD and clicked on the hint button. This resulted in the following message: "there is no obvious square that can be solved at present". I do not see any 'advanced' methods that apply (x wing, swordfish, etc.) except for one xy wing that does not advance the puzzle.
Is it possible that I have to resort to trial and error / guessing??
Any comments ? Click here to contributeI have had a look and it looks like one where you need to make a guess to make any progress.
All I can spot is that there is a Swordfish that knocks out 1 in square Cb that does not get you anywhere really.
Any comments ? Click here to contributeI entered the puzzle presented into my own analysis tool. Putting a 5 in the Ih cell blew the puzzle wide open. I solved it in a few minutes.
Any comments ? Click here to contributeI need a little help with this puzzle. Can't seem to find a move. Thank You - This puzzle has me stumped, what should I do next ?
Any comments ? Click here to contributeAgain, I entered this puzzle into my sudoku cruncher. It showed a three-number ambiguity in Row A which pretty much dictated a 3 in cell Aa.
I dropped a 3 in cell Aa and it blew the puzzle wide open. Solved in minutes
Any comments ? Click here to contributeIn the 'Theory' section of this web site, there is a section labeled 'Hardest Sudoku Puzzle'. There are two puzzles given in the section. The second one seems fairly straightfoward to solve. The first one however seems quite difficult (to me at least).
Does anyone know if it can be solved without backtracking? If so, can you tell me what strategies work with it, at least to solve the first cell?
Any comments ? Click here to contributeI agree the one with a big gap in the middle is fairly easy but the other one is diabolical. As you say not one square is easy to solve. I haven't looked for Alternate Pairs but that is the only strategy I didn't try...
Any comments ? Click here to contributeI tried the 'Hardest Sudoku Puzzle' about halfway down the web page Sudoku Puzzle Theory just to see how hard it really is.
YES! it is VERY hard. I was unable to get ANYTHING solved - not even able to eliminate even a single candidate.
I am moderately experienced, but because the number of possibilities is so large I am unable to sort through the zillions of possibilities in trying to locate Swordfishes and even 4 or 5 Swordfish chains. (I don't use a program to help me - seems like cheating to me.)
Can someone suggest and explain the first step in this puzzle. (If possible keep the explanations in simple terms.)
Any comments ? Click here to contributeNo I can see no way to make even one move.
I reckon that someone must have generated it just as an example of a hard puzzle rather than one that can be solved with 'standard' techniques.
Any comments ? Click here to contributeAnyone know how to proceed with solving this?? some advanced method? Thanks
Any comments ? Click here to contributeI had a long look at this and agree that I can't see a move.
But when I got SudokuDragon to try and help me, it reckoned it is a bad puzzle?!
It claims there is more than one solution, which could explain things.
Where did you get the puzzle from - is it generated by SudokuDragon?
Any comments ? Click here to contributeHave fun solving!! - This puzzle requires some interesting use of strategy
Any comments ? Click here to contributeThis puzzle shows error when I enter the only possibility in the cell, yet when I use Hint/Show me incorrect allocation, no allocations are shown.
Any comments ? Click here to contributeYes, there is a problem with this puzzle.
When I open your puzzle with the dragon and use the Hint 'Check whether all allocation are correct' it tells me that there are problems - the puzzle can not be solved.
If I go back through the allocations made it is the allocation of 6 in Bf that seems to be the problem.
If I undo to this point and use View | Advanced Exclusions it shows that Bf can not take a 6 due to Alternate Pairs. The pattern of 6s as possibilities that prevent this are squares Ba; Db; Df; and Ea. These combine to disallow a 6 in Bf - it should be allocated a 1.
If you would prefer SudokuDragon not to allow you to make incorrect assignments then you can do this by selecting the 'Warn me when I make incorrect allocations' on the Options tab (within View Options).
Once you make an incorrect allocation all bets are off as to where possibilities will lead you as it is not a valid puzzle with a unique solution any more.
Any comments ? Click here to contributeI have used all the basic strategies including a couple of x-wing applications. At this point a choice a Cf will either win or lose. Is there a way of analyzing the position without flipping a coin? I am a relative newbie, so I am probably just missing something. Thanks!
Any comments ? Click here to contributeYes this is quite a tricky one to work out without guessing.
Actually SudokuDragon does show the way, if you use the 'Advanced exlusions' then it will indicate that a number of squares can be solved using alternate pair analysis.
Concentrate just on where '5's can go by selecting '5' in the toolbar.
This shows that there is an X-Wing in the puzzle Cf; Ch; Hf and Hh give a box and this excludes 5s in Ha; Hc; Hd and Hg for row H and Cc for row C.
One more tricky one, column g has only two squares that can take 6 or 7 Bg and Cg so the hidden pair rule stops a 3 going in Bg and more importantly in Cg preventing a 3 or a 5 going there.
These have created an alternate pair in column g as there are now only two squares a 5 can go (Ag and Ig). Now take out your coloring pens.
Cg is Orange and Ch is Green this requires Hh to be orange and Hf green (this was the X-Wing previously discovered). Now Hh links to Ig as another pair so Ig is green. Our new pair in column g allows us to color Ag orange. The pair in row A links to set Ac green. From Cf the pair in region Ad sets Bd green and from column d Gd to orange.
That's it, and now it is time to look at the pattern. There is now a green square in row B and row I. But there is an uncolored pair in column b with one square Bb and the other Ib. A 5 must go in one of those squares but both are incompatible with the green squares. This therefore requires that 5 go in all the orange squares (Ag Cf Gd Hh).
Then the puzzle can be solved without a guess!
Any comments ? Click here to contributeI have tried alternate pair analysis and have eliminated a couple of numbers, but not enough to solve the puzzle. I'm relatively new to Sudoku so there could definitely be something I have missed.
Thanks.
Any comments ? Click here to contributeYou are right that alternate pairs play a key part in solving this one.
If you look at the 2s you will see there are 5 pairs in all.
I have marked the useful ones in red and blue.
Da and Ha are in column a; Ha links to Gc in region Ga and then Gc links to Gg in row G.
This is very useful because Dg is at the intersection of row D and column g.
If the 2 goes in the red squares then a 2 can not go in Dg. Conversely if a 2 goes in the blue squares a 2 can not go in Dg. So a 2 in not possible at all in Dg. That means a 4 MUST be allocated in Dg. All the remaining
squares can then be easily solved.
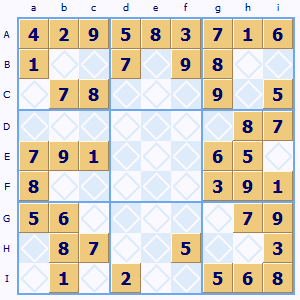
Any comments ? Click here to contributeLong time fan of Sudoku, new user of Dragon. I was looking for strategy help. I feel like I understand the concepts necessary for the beginer to moderate puzzles, but quickly get stumped when there are too many possibilities. I am sharing a specific puzzle to illustrate where I get blocked and would like to understand how get over this hurdle and in general what is the concept I am missing and how to use Dragon to learn it. I have marked possibilities, i hope those come through.
The hint tells me that Ac has to be a 3 and that 7 is excluded because it is forced to occur in Row C. I get that Column b is down to 2 possibilities, but since they occur across a region boundary I don't see how that helps me. Isn't a 7 just as likely to be in Db as in Cb?
Looking one more step forward, the possibilities in Cb are narrowed to 7, excluding 8 because of Alternate pair colouring? I don't follow that either.
thank you in advance.
Any comments ? Click here to contributeI think this is quite a challenging puzzle. The next square to solve seems to require alternate pair reasoning [See the page on alternate pairs].
If you mark the 8s looking at pairs you should end up with this pattern of squares:
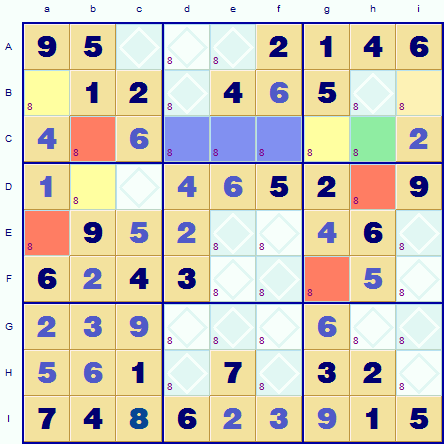
The crucial point is that squares Ca and Cg are the only two squares that can take an 8 in row C and these form an alternate pair.
The squares in blue are excluded due to the shared sub-group rule, as an 8 can only be in Ad or Ae in the Ad region (can't go anywhere else in row A). Square Ch is the hard one as it involves spotting a four member hidden group for 1,3,5,9 - there are only four squares in row C that can take these numbers. This means both 7 and 8 are excluded from Cd; Ce; Cf and Ch.
Having worked out these restrictions Cg is now part of an alternate pair for 7s with Cb and this is paired with Fg. Now there are two red squares in region Dg which means using the alternate pair logic 7 can not go in the red squares it must go in all the yellow ones. This lets 3 squares be solved Ba; Db and Cg. All the remaining squares are then straightforward.
Sudoku Dragon can help with this is you dswitch on display of Automatic possibilities with the highlighting set to 'Advanced Exclusions', even so it is hard to follow.
Any comments ? Click here to contributeCopyright © 2005-2024 Sudoku Dragon