Puzzles that have people stumped
Puzzles from contributors looking for help and advice. These tend to be somewhat easier puzzles.Seeking advice on how best to solve a gentle or moderate puzzle.
Contributors have uploaded puzzles for you to look at and help them to solve.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeJust started some harder puzzles and I'm stuck. tried having
sudoku dragon give me some advice but it said no obvious solution ...at least in easy mode and I couldn't figure how to change the mode. here's the puzzle anyway ..hope it shows up
Thanks Steve
 Any comments ? Click here to contribute
Any comments ? Click here to contributeYes you need to look beyond thge 'only choice' and 'only square' rules to solve this.
To get SudokuDragon to show more excluded possibilities you need to use the 'highlight' options on the View menu. Choose 'forced allocations' for easier squares and then 'Exclusions' to include 'twin' and 'shared subgroup' exclusion. If that does not help you can get further help by selecting 'Advanced Exclusions' which brings in Alternate Pair strategy into play.
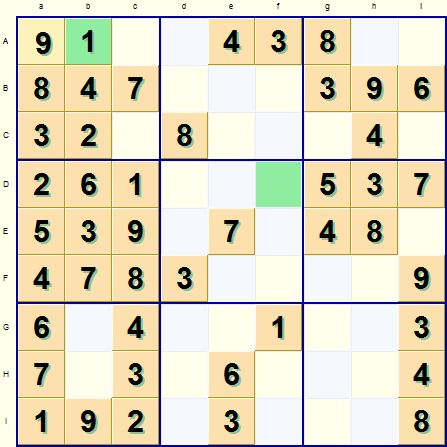
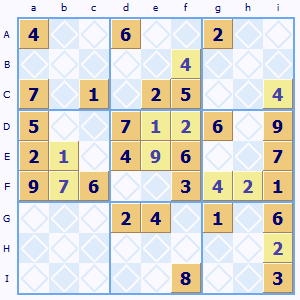
In this particular puzzle, all I think you need is the 'shared exclusion rule'.
Row B lacks a 1; 2 and 5 to complete it. All these squares are shared by region Ad that all means that the 1;2 and 5 can ONLY be in row B.
So the 2 and 5 in Ad can be knocked out. Also 1 and 5 in Ce and finally 5 in Cf.
As that only leaves 9 as a possibility for square Ce, a 9 must go in that square - that should help get you going.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeI am a beginner, can you tell me how to get started on this one?
 Any comments ? Click here to contribute
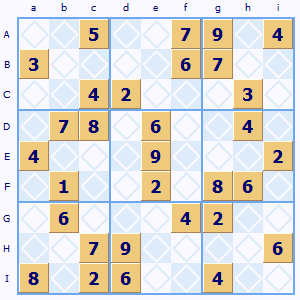
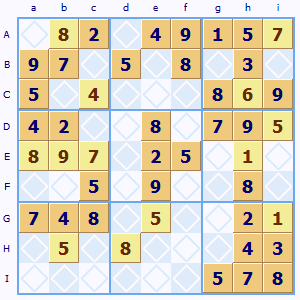
Any comments ? Click here to contributeWell Geraldine, the most important sudoku strategy to learn to start with is the two out of three rule.
What you do is look through all the rows and then all columns looking for each number in turn and look for rows in which you get 2 rows or columns out of three that have already been solved.
In this case scan the 1s by rows - this does not help as there is only one 1 in square Fb.
Now look at the 2s, no luck in rows A;B;C but there is in row E (Ei) and F (Fe) these two 2s in a group of three rows mean a 2 must occur in row D and it's also certain that it must be in column a;b; or c. Only Da is free so the 2 must go in Da. Next look at the rows G;H;I there is a 2 in Gg and Ic so once again it can be
proven that the missing 2 in row H must be in column d;e; or f. Now it can't go in He because of the 2 in Fe so the only square left in this row it can go in is square Hf. That's 2 squares solved.
Continue looking through the rows, this time for 3s. In rows A;B;C there are two 3s in Ba and Ch so the 3 must be in Ad or Ae. At this time it can't be determined which of the two it is, this often happens. There are no more 3s in the remaining rows so can move on to 4s.
With 4s in rows A;B;C there are two once again in Ai and Cc restricting the choice to Bd or Be - can't quickly determine which. Now with rows D;E;F you sport two in Dh and Ea so that leaves a 4 to go in Fd or Ff. Because of the 4 in Gf it cant go in Ff so it must go in Fd.
I think that's enough to show the general two out of three strategy in action. You continue with rows going throughh 5;6;7;8 and finally 9. When that is done you do the columns in just the same way working through the numbers 1 to 9.
If you solve any squares that means you might find more to solve the next time around - you get to be able to do the scan very quickly and what is wonderful is that you do not need to write anything down - you can do it in your head - and that really impresses people.
 Any comments ? Click here to contribute
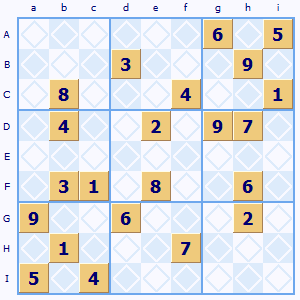
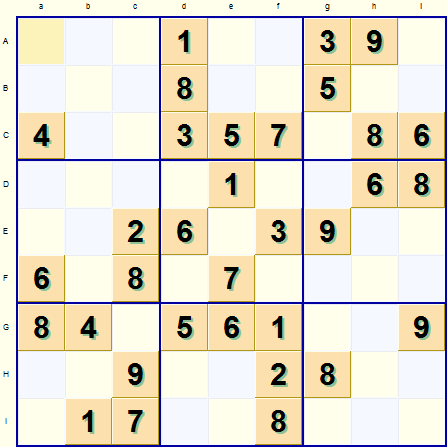
Any comments ? Click here to contributeThis puzzle has me stumped. What should I do next to try and solve it ?
 Any comments ? Click here to contribute
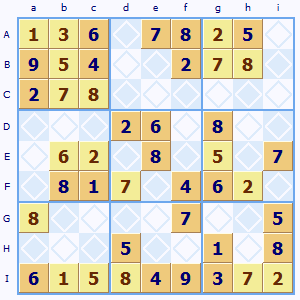
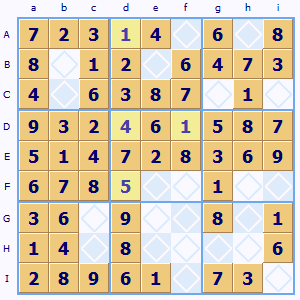
Any comments ? Click here to contributeAs far as I can see there are no obvious to squares to solve first.
I tend to look for nearly complete groups - (rows, columns or regions). In this case row A and column g are both missing two squares. The two symbols missing in both cases happen to be '4' and '9'. If you use the SudokuDragon possibility bar and click on '4' you will see that it is going to be critical as to where the '4' is allocated. Use the same techique for '9'.
There is a chain of reasoning here. If '9' rather than '4' goes in Ad then that forces a '9' in Fe as it is the only square in column e it can go. If now look for allocating in row E a '9' must go in Eh as it is the only square that can take a '9' now.
The region Ag is now crucial as to where the '9' can go - only two squares are possible Cg and Ci. But it can't go in Cg because row i has only one place for a '9' Ci. So it looks like Ci is the place - but this is where the clinch is made. The symbols '1';'3' and '6' must occur in the region Ag in the squares Bi Ch and Ci. This forms a triplet and it means '9' can not go in Ci. So this route has failed the '9' must and can not go in Ci so the initial assumption of '9' in Ad must be wrong. The '4' must be the correct choice for Ad. After that it must be plain sailing.
Download the puzzle and see if you can spot any easier strategy than that.
 Any comments ? Click here to contribute
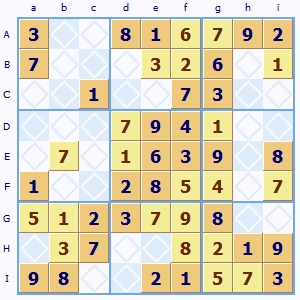
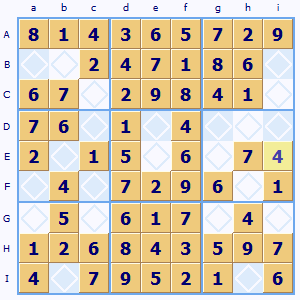
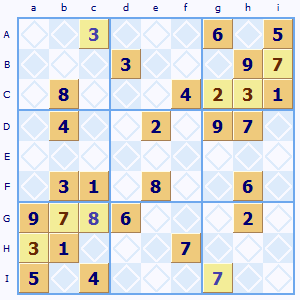
Any comments ? Click here to contributeI am stuck. Please help. I have solved all the easy squares and can not find
one that I can see that is easy to solve. What is the best strategy to apply (puzzle attached for you to download and look at).
 Any comments ? Click here to contribute
Any comments ? Click here to contributeAlthough there are no obvious allocations as far as SudokuDragon is concerned there are two squares that are fairly easy to solve and should provide the key to solving the complete puzzle.
The squares I have spotted are Bb and Ac. Row A is crucial, there are only a '4' and '5' missing in this row so '4' and '5' must be in Ab and Ac and therefore they can not be in any other square within the region - especially Bb. For Bb the only other possibility is a '9' so a '9' must be allocated in Bb. Also the square Ac must be either a '4' or '5' and nothing else. However there must be a '4' in column b but the only squares a '4' can go are Ab; Bb or Cb. Because it must be in one of these '5' can't got in Ac so Ac is forced to be a '5'.
Hope this helps.
 Any comments ? Click here to contribute
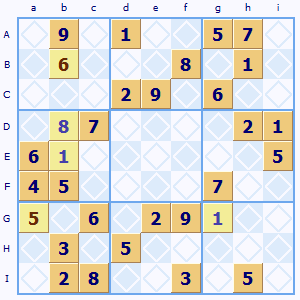
Any comments ? Click here to contributeHelp please, I'm stuck - again...
 Any comments ? Click here to contribute
Any comments ? Click here to contributeThe key square to look at is Gd. There seem to be only two possibilities 5 or 9.
But the 9 can not go there. In Ad only 3 and 9 are possible, but this also the case for Ed. This is an example of a 'naked' twin (see strategy page) so both 3 and 9 can not possibly go elsewhere in column d. So that means Gd must be a 5.
There is also the Sudoku stripe to look out for. In this puzzle it is located in row F in reverse order. That should certainly get the puzzle sorted.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeYou may not agree with the way I do my sudokus, but for what it is worth, I can tell you how I solved this one.
First, I loaded the puzzle into my sudoku cruncher (personally developed). I observed that in the top center sector, there are only two places for a number 1... cell Af and cell Bd. I opted to use cell Bd. That only gave me three additional cells.
Then in Row A, this leaves only two places for a number 7... cell Ae and Af. I used cell Af. that fills 6 additional cells.
Next the bottom-center sector is left with a 7,6 twin in cells He and Ie. I placed a 7 in cell Ie. That blew it wide open and I went on to a total solution in a few minutes.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeThe easy answer once again is to look for the Sudoku Stripe!
Once you locate this you can solve the puzzle without much difficulty.
There is only one place that the stripe could be located in this sudoku puzzle. It is in column f in reverse order. From what I can see once you fill in the numbers 1 to 4 and 6 to 7 in the stripe all the remaining squares are fairly easy to solve.
 Any comments ? Click here to contribute
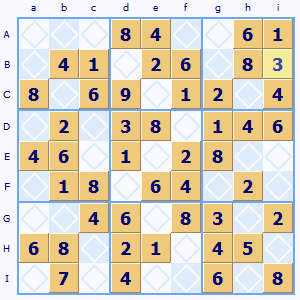
Any comments ? Click here to contributeBe patient, please, I am a newbie. I don't know where to go next with this. I also need help understanding the terms "group rule" and "shared sub-group". Where can I find these definitions? And how/where can I learn some advanced stratagies?
 Any comments ? Click here to contribute
Any comments ? Click here to contributeAnybody help please. What strategy might take this further? Thanx.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeThis might a little but not clinch it.
There are quite a few chains in the puzzle that let you knock out some of the 3s.
In column a there is a 5;9 3;5 3;9 chain that eliminates 3 from Ba.
Next door in column b there is a 3;9 8;9 3;8 chain that eliminates 3 from Eb.
In region Ga there is another 3:9 8:9 3:8 chain that eliminates 3 from Gc.
I also note that if you are forced to guess it's a question of a '2' in either Dg or Di, but I'd hate to resort to that.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeI think your best bet is to look at the 8s for this puzzle.
When I get stuck near the end of a puzzle I scout through all the numbers with a few squares left (not too many like 3 or too few like 2 in this case).
Only the 8s look promising and the way I work it is to mentally work through an either or case. Look at center region with only De or Ee as possibilities. If an 8 went in De then this means an 8 must go in Eb this is because Fc could not be an 8 as Fh is the only square in Dg that can now take an 8. That then forces an 8 in Gc. But that's impossible because theree is now no square in region Gg that can take an 8 any more. Ih can't because of the 8 in Fh and can't in Gi either. So the initial test for De as 8 was wrong.
Therefore it must be allocated in Ee not De...
Some clever person will no doubt spot this as a Swordfish scenario which precludes De but I'm no expert just a plodder... There are certainly four rows with only 2 8s in them and I think that discounts De and Dh.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeEven though there are 2 singles in this puzzle, I have solved 6 more cells and am at a sticking point. This is a tuff one!! What strategies can be applied?
 Any comments ? Click here to contribute
Any comments ? Click here to contributeLooks like this is the start state of the puzzle rather than when you got stuck.
Anyway, here's the first few squares I managed to solve
Ch has to be a 3 as there is no other choice for the square.
Gc has to be a 7 - also only choice
More tricky one - Ac must be a 3 - there are only two squares in row A it can go Aa and Ac but it can't go in Aa because of the twin 1,4 in squares Aa and Ab (only squares that 1,4 can go).
This knocks out a possibility of 3 in Gc and this must now be an 8.
Also in this region Ha must be a 3 as that is the only square that can take it.
Now in Row H a 7 can go in Ig or Ii, But actually Ii is not possible as 6 and 9 must go in either Hi or Ii so it can't be a 7 here.
That knocks out a 7 in Cg so that must now be a 2. Looking in the region Ag the square Bi must now be a 7.
Does that help at all?
 Any comments ? Click here to contribute
Any comments ? Click here to contributeI loaded the puzzle into my sudoku cruncher. Initially, the only two givens are the two cells mentioned by Mr. Wood.
I then looked for a sector that has a number with only two cell possibilities. I placed a 9 in cell Eb. That led to a complete solution.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeThis daily puzzle has me stuck
 Any comments ? Click here to contribute
Any comments ? Click here to contributeI must admit to have been a little stuck to begin with.
There doesn't even seem to be an X-Wing to help out!
I then realised that I hadn't looked for the Sudoku stripe that is present in all the daily puzzles.
It takes a little spotting but there it is in reverse order in row D. This takes in part of the central region so the rest of the puzzle should be easy to solve.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeI, nor SD, has an obvious next step. I'd like to see a 'next cell' and why...TIA.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeThere is a fairly straightforward answer I think.
I wonder what settings you are using on SudokuDragon, if I switch on 'Advanced Exclusions' on the 'View' menu I get several squares suggested. For example is shows that a '4' can not go in 'Bd' so a '1' must go there. This is due to the Shared square rules. There are lots of possibilities that can be excluded due to either the hidden/naked group rule or the shared group rules.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeI would like to solve this puzzle.
 Any comments ? Click here to contribute
Any comments ? Click here to contributeIt took a while to sopt the one square that unlocks the puzzle, but with that one square the puzzle becomes easy to solve.
There are only '3's and '7's left in any numbers to allocate.
Fortunately in column b there are only two possible squares a 3 can go, and they are both in region Aa. This means that a 3 can not go in Ba, because it must be in Ab or Cb - and in both cases it cant go in Ba.
Now with a '3' not possible in Ba this forces a '3' to go in Bi as that is the only remaining possiblity for a '3' in row B.
Allocating a '3' here unlocks everything a '7' must now go in Ch etc...
 Any comments ? Click here to contribute
Any comments ? Click here to contributeStumped me!! - This puzzle has me stumped, what should I do next ?
 Any comments ? Click here to contribute
Any comments ? Click here to contributeCopyright © 2005-2025 Sudoku Dragon